Integration of sec square x | Integral of sec^2x

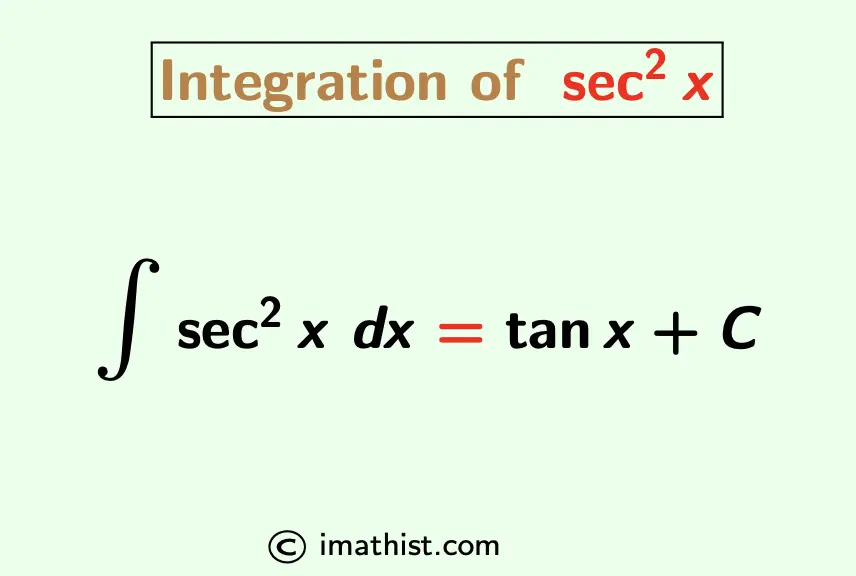
The integration of sec square x (sec²x) is equal to tanx+C. In this post, we learn how to integrate sec^2x.

Sec^2x Integration Formula

The integration formula of sec²x is given by

∫sec²x dx = tanx +C

where C denotes an integral constant.

What is the Integration of sec^2x

Answer: The integration of sec^2x is tanx+C.

Proof:

First, recall the function whose derivative is the function sec²x. We know that the derivative of tanx is equal to sec²x, that is,

$\dfrac{d}{dx}$(tan x) = sec²x.

Integrating both sides, we get

∫$\dfrac{d}{dx}$(tan x) = ∫sec²x dx +K, K is an integral constant.

⇒ tan x = ∫sec²x dx +K

[this is because the integration is the opposite process of derivatives]

⇒ ∫sec²x dx = tanx -K

⇒ ∫sec²x dx = tanx +C where C=-K.

So the integration of sec²x (sec square x) is equal to tanx+C where C is an arbitrary constant of integration.

Related Derivatives:

Integration of sin2x	Integration of sin3x
Integration of cos2x	Integration of cot2x
Integration of tan2x	Integration of tanx

FAQs