Derivative of lnx: Formula, Proof by First Principle, Chain Rule

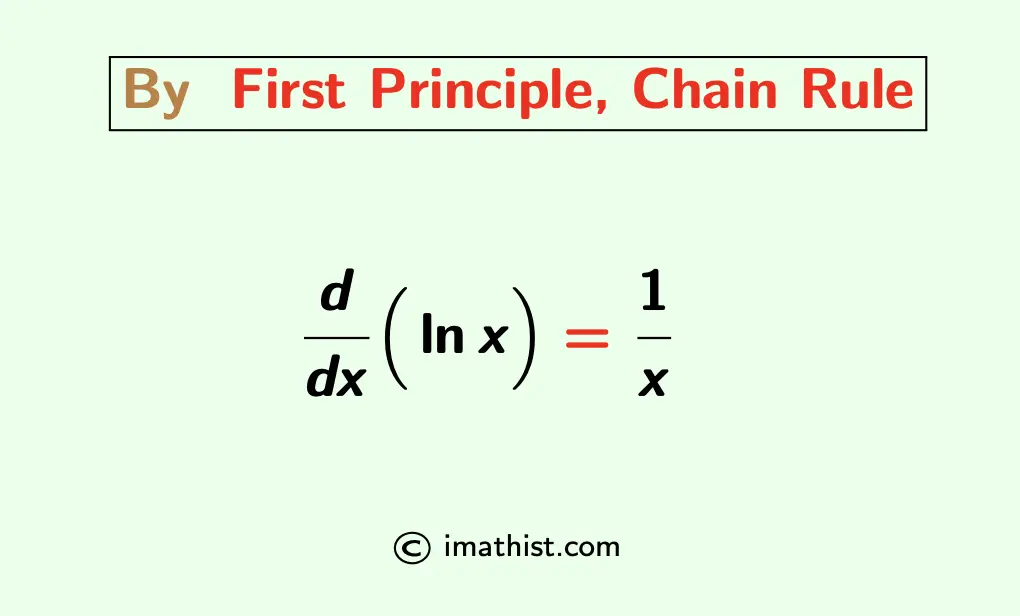
The derivative of lnx is equal to 1/x. Ln(x) denotes the natural logarithm of x, that is, lnx= log_ex. Here we will find the derivative of ln(x) using the limit definition and chain rule of differentiation.

Note that lnx= log_ex. The derivative of lnx is denoted by d/dx(lnx), and its formula is given as follows:

$\dfrac{d}{dx}(\ln x)=\dfrac{1}{x}$.

Find the Derivative of lnx

Answer: The derivative of lnx is 1/x.

Explanation:

Let z= lnx

⇒ z = log_ex

⇒ e^z = x

Differentiating both sides we get

$e^z \dfrac{dz}{dx}=1$, obtained by the chain rule.

⇒ $e^{\ln x} \dfrac{dz}{dx}=1$

⇒ $x \dfrac{dz}{dx}=1$ as we know e^lnx =x.

⇒ $\dfrac{dz}{dx}=\dfrac{1}{x}$

That is, $\dfrac{d}{dx}(\ln x)=\dfrac{1}{x}$.

Therefore, the derivative of lnx is equal to 1/x, and this is obtained by the chain rule of differentiation.

Also Read:

• Derivative of 1/lnx
• Derivative of ln u
• Derivative of ln 3x

Derivative of lnx by First Principle

Let us recall the first principle of derivatives.

The derivative of a function f(x) by first principle is given by the following limit formula: $f'(x)$ = limh→0 $\dfrac{f(x+h)-f(x)}{h}$.

Put f(x) = lnx.

So (lnx)$’$ = lim_h→0 $\dfrac{\ln (x+h)-\ln (x)}{h}$

= lim_h→0 $\dfrac{\ln \Big(\dfrac{x+h}{x} \Big)}{h}$, by the difference rule of logarithms.

= lim_h→0 $\dfrac{\ln \Big(1+\dfrac{h}{x} \Big)}{h}$

Let h/x = z, so that z→0 when h→0. Also, h=zx.

= lim_z→0 $\dfrac{\ln \big(1+z \big)}{zx}$

= $\dfrac{1}{x}$ lim_z→0 $\dfrac{\ln \big(1+z \big)}{z}$

= $\dfrac{1}{x} \times 1$, using the limit formula: lim_x→0 ln(1+x)/x =1.

= 1/x.

So the derivative of lnx by first principle is equal to 1/x.

More Reading:

• Derivative of x^lnx
• Derivative of ln(ln x)
• Derivative of log(sin x)
• Derivative of root logx

FAQs