Derivative of sec2x by First Principle

The derivative of sec2x by first principle is equal to 2sec2x tan2x. In this post, we will find the derivative of sec2x by first principle. The first principle rule of derivatives states that the derivative of f(x) is given by the limit

f$’$(x) = lim_h→0 $\dfrac{f(x+h)-f(x)}{h}$.

Let us now find the differentiation of sec2x by first principle.

Derivative of sec2x from first principle

To find the derivative of sec2x using first principle, let us put

f(x)= sec 2x

in the above limit formula. So the derivative of sec2x will be equal to

(sec2x)$’$ = lim_h→0 $\dfrac{\sec 2(x+h)-\sec 2x}{h}$

= lim_h→0 $\dfrac{1}{h}\Big[\dfrac{1}{\cos(2x+2h)}-\dfrac{1}{\cos 2x}\Big]$ as we know that secx=1/cosx.

= lim_h→0 $\dfrac{1}{h}\Big[\dfrac{\cos 2x -\cos(2x+2h)}{\cos (2x+2h)\cos 2x}\Big]$

= lim_h→0 $\dfrac{1}{h}\Big[\dfrac{2\sin \frac{4x+2h}{2}\sin \frac{2h}{2}}{\cos (2x+2h)\cos 2x}\Big]$ using the formula cosa- cosb = 2sin(a+b)/2 sin(b-a)/2

= lim_h→0 $\dfrac{1}{h}\Big[\dfrac{2\sin(2x+h) \sin h}{\cos (2x+2h)\cos 2x}\Big]$

= $\dfrac{2\sin(2x+0)}{\cos (2x+0)\cos 2x}$ lim_h→0 $\dfrac{\sin h}{h}$

= $\dfrac{2\sin 2x}{\cos 2x \cos 2x} \cdot 1$, this is because lim_x→0 (sinx/x) =1.

= 2 sec2x tan2x as we know tanx=sinx/cosx.

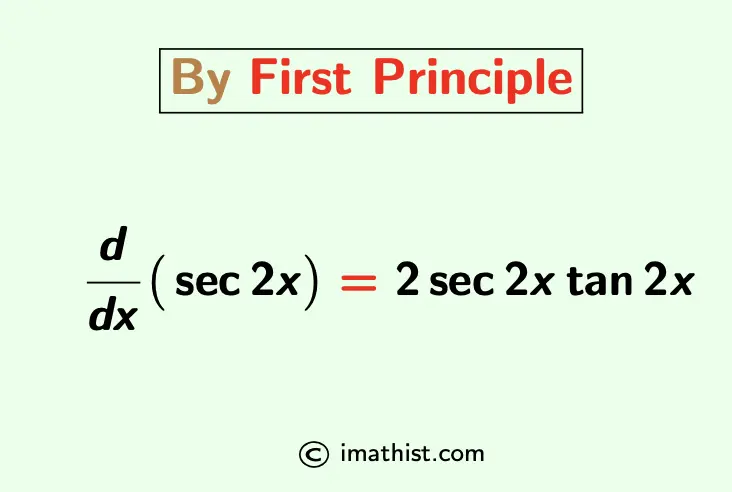
So the derivative of sec2x is equal to 2 sec2x tan2x and this is obtained by the first principle of derivatives.

The derivative formula of sec2x is given by

$\dfrac{d}{dx} \big(\sec 2x \big)= 2\sec 2x \tan 2x$.

ALSO READ:

• Derivative of root x by first principle
• Derivative of tan²x by first principle
• Derivative of $\sqrt{\sin x}$ by first principle
• Derivative of $\sqrt{\cos x}$ by first principle
• Derivative of |x|

FAQs