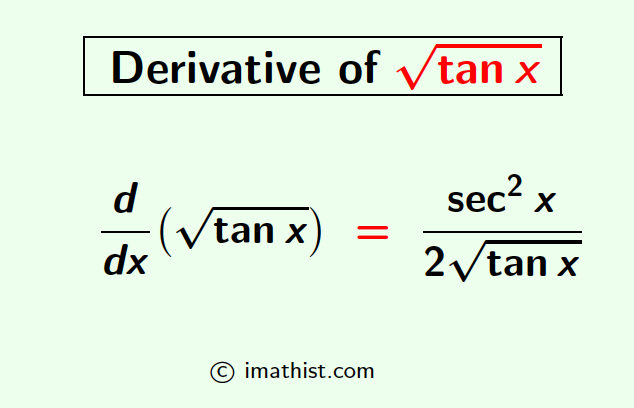
The derivative of root tanx is equal to sec2x/(2√tanx). In this post, we will learn how to differentiate the square root of tan x using the first principles.
Derivative of square root of tanx from first principle
Question: Find the derivative of $\sqrt{\tan x}$ from first principle.
Solution:
The below steps have to be followed to find the derivative of square root of tanx using first principle.
Step 1:
Let f(x) = $\sqrt{\tan x}$
We know that the derivative of f(x) by first principle is given by
$\dfrac{d}{dx}(f(x))$ $=\lim\limits_{h \to 0} \dfrac{f(x+h)-f(x)}{h}$.
Step 2:
Putting f(x)=$\sqrt{\tan x}$ in the above limit, we get that
$\dfrac{d}{dx}(\sqrt{\tan x})$ $=\lim\limits_{h \to 0} \dfrac{\sqrt{\tan (x+h)}-\sqrt{\tan x}}{h}$
We will multiply both the numerator and the denominator by √tan (x + h) + √tan x. By doing so, the above
= $\lim\limits_{h \to 0} \Big[ \dfrac{\sqrt{\tan (x+h)}-\sqrt{\tan x}}{h}$ $\times \dfrac{\sqrt{\tan (x+h)}+\sqrt{\tan x}}{\sqrt{\tan (x+h)}+\sqrt{\tan x}} \Big]$
= $\lim\limits_{h \to 0} \dfrac{(\sqrt{\tan (x+h)})^2-(\sqrt{\tan x})^2}{h(\sqrt{\tan (x+h)}+\sqrt{\tan x})}$, this is obtained by using the formula (a-b)(a+b)=a2-b2.
= $\lim\limits_{h \to 0} \Big[ \dfrac{\tan (x+h)-\tan x}{h}$ $\times \dfrac{1}{\sqrt{\tan (x+h)}+\sqrt{\tan x}} \Big]$ …(∗)
Step 3:
Now, by the first principle of derivatives, the limit $\lim\limits_{h \to 0} \dfrac{\tan (x+h)-\tan x}{h}$ $=\dfrac{d}{dx}(\tan x)$ = sec2x.
So applying the product rule of limits, we deduce from (∗) that
$\dfrac{d}{dx}(\sqrt{\tan x})$ = $\lim\limits_{h \to 0} \dfrac{\tan (x+h)-\tan x}{h}$ $\times \lim\limits_{h \to 0} \dfrac{1}{\sqrt{\tan (x+h)}+\sqrt{\tan x}}$
= sec2x × $\dfrac{1}{\sqrt{\tan (x+0)}+\sqrt{\tan x}}$
= sec2x × $\dfrac{1}{2\sqrt{\tan x}}$
= $\dfrac{\sec^2 x}{2\sqrt{\tan x}}$
Thus, the derivative of square root of tanx is equal to sec2x/(2√tanx) and this is obtained from the first principle of derivatives.
Related Topics:
Derivative of $\sqrt{\sin x}$ from first principle
Derivative of $\sqrt{\cos x}$ from first principle
Derivative of $\sqrt{\sin x}$ by chain rule
Derivative of $\sqrt{\cos x}$ by chain rule
FAQs
Q1: What is the derivative of root tanx?
Answer: The derivative of square root tanx is equal to sec2x/(2√tanx).